
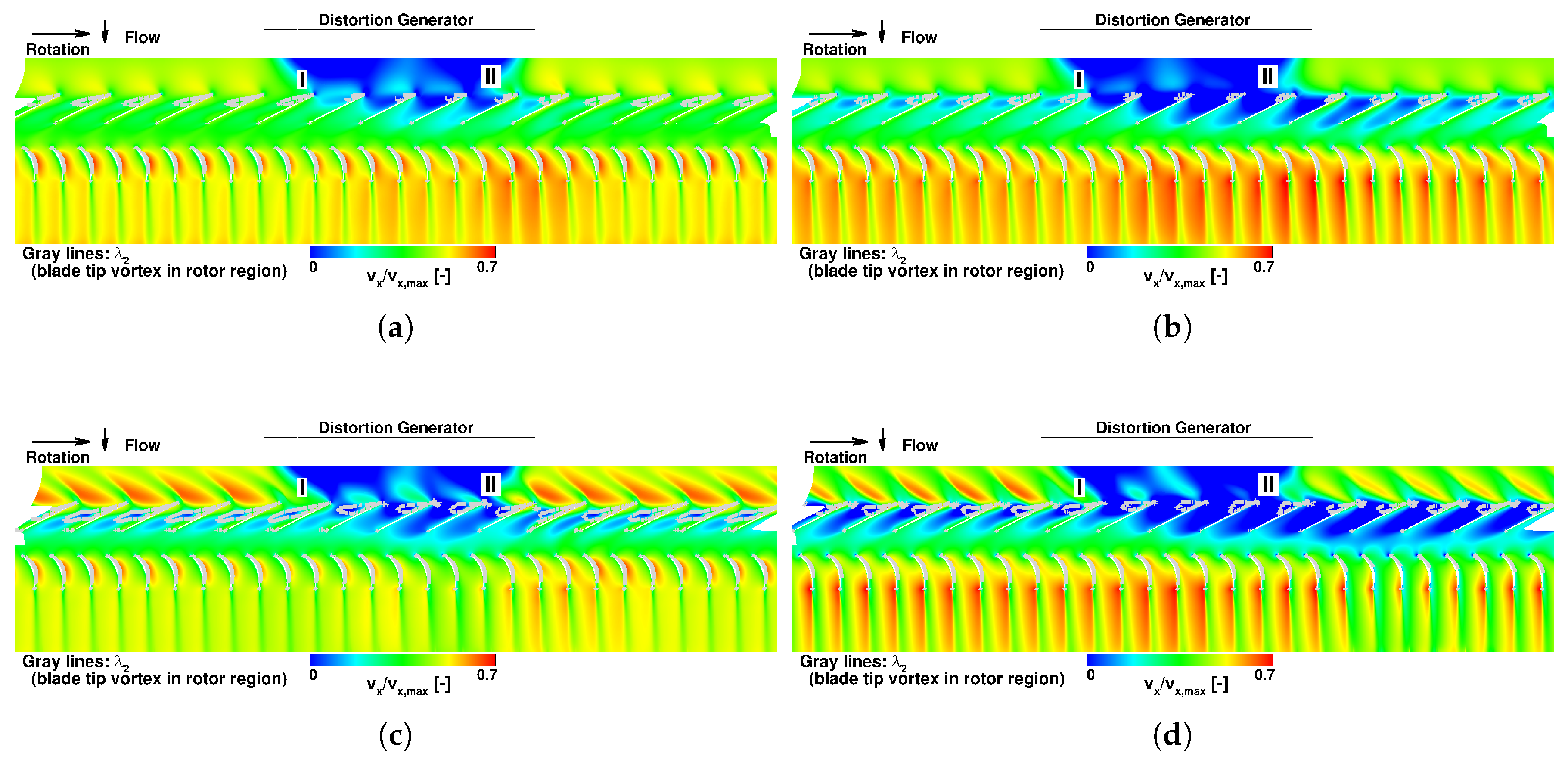
This pressure difference, applied to the surface area with proper regard to force direction, gives a net lifting force. Thus, in accordance with the principle of conservation of energy, the mean static pressure must be less on the upper surface than on the lower surface. If the path from front to rear stagnation point is longer along the upper surface than along the lower surface, the mean velocity of flow along the upper surface must be greater than that along the lower surface. The two points of zero local velocity are known as stagnation points. Air approaching the leading edge comes to rest at some point on the leading edge, with flow above this point proceeding around the upper airfoil surface to the trailing edge, and flow below passing along the lower surface to the same point, where the flow again theoretically has zero velocity.
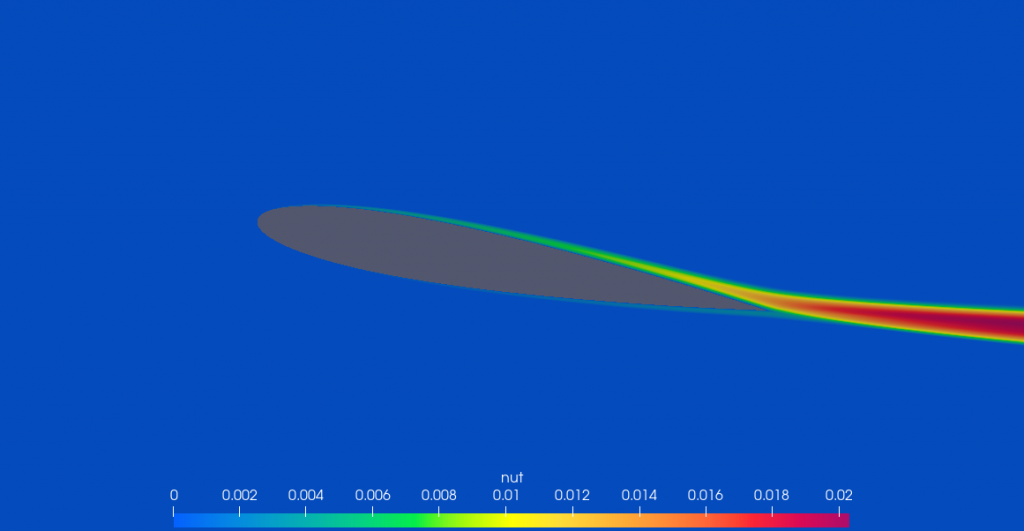
See Transonic flightĪ typical subsonic wing cross section (airfoil) has a rounded front portion (leading edge) and a sharp rear portion (trailing edge). The theories which apply to compressible, inviscid fluids may be used almost without modification in some low-subsonic problems, and in other cases the results offered by these theories may be modified to account for the effects of viscosity and compressibility. In both cases the viscosity of the fluid is important. Subsonic flow of a fluid such as air may be subdivided into a range of velocities in which the flow may be considered incompressible without appreciable error (below a velocity of approximately 300 mi/h or 135 m/s), and a higher range in which the compressible nature of the fluid becomes significant. The design of each is affected by the same principles of subsonic aerodynamics. Vehicle type may range from a small helicopter, which operates at all times in the lower range of the velocity scale, to an intercontinental ballistic missile, which is operative throughout this and other velocity regimes, but is in subsonic flight for only a few seconds. At higher vehicle velocities the local velocity of air passing over the vehicle surface may exceed sonic speed, and true subsonic flight no longer exists. Subsonic flight extends from zero (hovering) to a speed approximately 85% of sonic speed corresponding to the ambient temperature. Movement of a vehicle through the atmosphere at a speed appreciably below that of sound waves. McGraw-Hill Dictionary of Scientific & Technical Terms, 6E, Copyright © 2003 by The McGraw-Hill Companies, Inc.


 0 kommentar(er)
0 kommentar(er)
